Zernike Polynomials¶

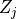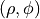The Zernike polynomials $Z_j$ are a complete sequence of polynomials that are orthogonal on the unit disk. Using polar coordinates $(\rho, \phi)$ , so that $(x, y) = \rho(\cos \varphi, \sin \varphi)$ , the Zernike polynomials are defined as

$\begin{eqnarray*} Z_j(\rho, \varphi) = Z_n^m(\rho, \varphi) = R_n^m(\rho) Y_m^j(\varphi), \end{eqnarray*}$

with

$\begin{eqnarray*} R_n^m(\rho) & = & \sum_{k=0}^{(n-m)/2} (-1)^k \left ( \begin{array}{c} n-k \\ k \end{array} \right ) \left ( \begin{array}{c} n-2k \\ (n-m)/2-k \end{array} \right ) \rho^{n-2k} \\ Y_m^j(\varphi) & = & \left \{ \begin{array}{ll} \cos(m\varphi), & \mbox{if}\; m\geq0, \\ \sin(m\varphi), & \mbox{if}\; m<0 \end{array} \right . , \end{eqnarray*}$

and where the integer index pair 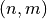$(n, m)$ is given by

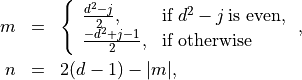
$\begin{eqnarray*} m & = & \left \{ \begin{array}{ll} \frac{d^2-j}{2}, & \mbox{if}\; d^2-j\,\mbox{is even}, \\ \frac{-d^2+j-1}{2}, & \mbox{if}\; \mbox{otherwise} \end{array} \right . , \\ n & = & 2(d-1) -|m|, \end{eqnarray*}$

where 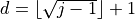$d = \lfloor \sqrt{j-1} \rfloor+1$ , and $\lfloor \cdot \rfloor$ represents the largest integer that is less or equal to the delimited integer.

Warning

Different orderings $(n, m) \rightarrow j$ of the Zernike polynomials are in use. Here, we followed [1] (page 213). Besides this, different scalings of the Zernike polynomials are used.

In the above, the Fringe convention as been used for scaling (c.f. http://en.wikipedia.org/wiki/Zernike_polynomials or http://mathworld.wolfram.com/ZernikePolynomial.html). For the sake of clarity, the following table lists the leading 36 (Fringe)-Zernike polynomials:

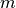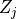$j$	$n$	$m$	$Z_j$	description
1	0	0	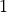$1$	piston
2	1	1	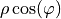$\rho \cos(\varphi)$	x-tilt
3	1	-1	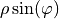$\rho \sin(\varphi)$	y-tilt
4	2	0	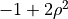$-1+2\rho^2$	defocus
5	2	2	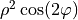$\rho^2 \cos(2\varphi)$	astigmatism
6	2	-2	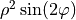$\rho^2 \sin(2\varphi)$	astigmatism
7	3	1	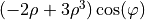$(-2\rho+3\rho^3) \cos(\varphi)$	coma
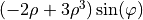8	3	-1	$(-2\rho+3\rho^3) \sin(\varphi)$	coma
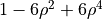9	4	0	$1-6\rho^2+6\rho^4$	spherical aberration
10	3	3	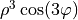$\rho^3 \cos(3\varphi)$	trifoil
11	3	-3	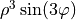$\rho^3 \sin(3\varphi)$	trifoil
12	4	2	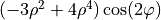$(-3\rho^2+4\rho^4) \cos(2\varphi)$	astigmatism
13	4	-2	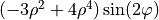$(-3\rho^2+4\rho^4) \sin(2\varphi)$	astigmatism
14	5	1	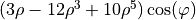$(3\rho-12\rho^3+10\rho^5) \cos(\varphi)$	coma
15	5	-1	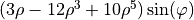$(3\rho-12\rho^3+10\rho^5) \sin(\varphi)$	coma
16	6	0	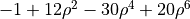$-1+12\rho^2-30\rho^4+20\rho^6$	spherical aberration
17	4	4	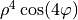$\rho^4 \cos(4\varphi)$	four wave
18	4	-4	$\rho^4 \sin(4\varphi)$	four wave
19	5	3	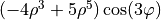$(-4\rho^3+5\rho^5) \cos(3\varphi)$	trifoil
20	5	-3	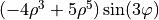$(-4\rho^3+5\rho^5) \sin(3\varphi)$	trifoil
21	6	2	$(6\rho^2-20\rho^4+15\rho^6) \cos(2\varphi )$	astigmatism
22	6	-2	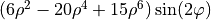$(6\rho^2-20\rho^4+15\rho^6) \sin(2\varphi)$	astigmatism
23	7	1	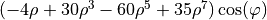$(-4\rho+30\rho^3-60\rho^5+35\rho^7) \cos(\varphi)$	coma
24	7	-1	$(-4\rho+30\rho^3-60\rho^5+35\rho^7) \sin(\varphi)$	coma
25	8	0	$1-20\rho^2+90\rho^4-140\rho^6+70\rho^8$	spherical aberration
26	5	5	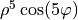$\rho^5 \cos(5\varphi)$	five wave
27	5	-5	$\rho^5 \sin(5\varphi)$	five wave
28	6	4	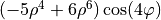$(-5\rho^4+6\rho^6) \cos(4\varphi)$	four wave
29	6	-4	$(-5\rho^4+6\rho^6) \sin(4\varphi)$	four wave
30	7	3	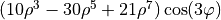$(10\rho^3-30\rho^5+21\rho^7) \cos(3\varphi)$	trifoil
31	7	-3	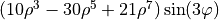$(10\rho^3-30\rho^5+21\rho^7) \sin(3\varphi)$	trifoil
32	8	2	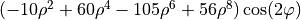$(-10\rho^2+60\rho^4-105\rho^6+56\rho^8) \cos(2\varphi)$	astigmatism
33	8	-2	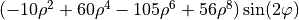$(-10\rho^2+60\rho^4-105\rho^6+56\rho^8) \sin(2\varphi)$	astigmatism
34	9	1	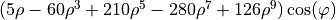$(5\rho-60\rho^3+210\rho^5-280\rho^7+126\rho^9) \cos(\varphi)$	coma
35	9	-1	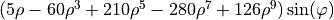$(5\rho-60\rho^3+210\rho^5-280\rho^7+126\rho^9) \sin(\varphi)$	coma
36	10	0	$-1+30\rho^2-210\rho^4+560\rho^6-630\rho^8+252\rho^{10}$	spherical aberration

Bibliography

[1]	Gross H. (editor), Handbook of Optical Systems, Volume III, Wiley-VCH 2005